Question

The SDMS student counsil held a bake sale. On thefirst day, the sold 14 cookies and 6 brownies for$32. On the second day, they sold 1 cookies and24 brownies for $86. Wht is the cost for each treat?Variables:

Answer 1

Variables: C = price of a cookie in dollars, B = price of a brownie in dollars

Equation for day 1 : 14c + 6b = 32

Equation for day 2: c + 2b = 86

Cookies cost $0.76 each and brownies cost $3.55 each

Step-by-step explanation:

Here, we want to get the cost of each of the treats

Let the price of cookies be $b and the price of brownies be $b

On the first day, they sold 14 cookies, which is a total of $14 * c = $14c , and 6 brownies which is $b * 6 = $6b

The sum of these two is $32. Mathematically, we have this as:

`\text{ 6b + 14c = 32}`

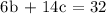
On the second day, 1 cookie ($c) and 24 brownies (24 * $b = $24b). The sum of these two is $86

We can write the equation as:

`\text{ c + 24b = 86}`

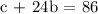
We have the two equations as follows:

`\begin{gathered} \text{ 14c + 6b = 32} \\ c\text{ + 24b = 86} \end{gathered}`

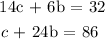
By using elimination, we multiply the first equation by 4 and the second by 1

We have this as:

`\begin{gathered} 56c\text{ + 24b = 128} \\ c\text{ + 24b = 86} \end{gathered}`

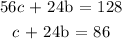
We subtract equation ii from i:

`\begin{gathered} \text{ 55c = 42} \\ c\text{ = }(42)/(55) \\ c\text{ = 0.76} \end{gathered}`

Finally, we get the value for a brownie by making a substitution into any of the two initial equations:

`\begin{gathered} \text{ c+ 24b = 86} \\ 24b\text{ = 86-c} \\ 24b\text{ = 86-0.76} \\ 24b\text{ = }85.24 \\ b\text{ = }(85.24)/(24) \\ b\text{ = }3.55 \end{gathered}`

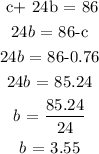
Now, let us fill in the answers as required by the question:

Variables: C = price of a cookie in dollars, B = price of a brownie in dollars

Equation for day 1 : 14c + 6b = 32

Equation for day 2: c + 2b = 86

Cookies cost $0.76 each and brownies cost $3.55 each