Answer:
Option D
Step-by-step explanation:
The exponential equation to determine the age of any radioactive object is as follows -
![t = [(ln(N)/(N_(0) ) )/(-0.693) ]* t_{(1)/(2) }](https://img.qammunity.org/2017/formulas/biology/high-school/zkil92my2ikv6nih88uj02goq33nkvziri.png)
where
 Final quantity of isotope after degradation
Final quantity of isotope after degradation
 initial quantity of isotope
initial quantity of isotope
 half life period of isotope
half life period of isotope
 time period of degradation
time period of degradation
Substituting the given values in above equation we get -
![4.6 = [(ln(N)/(N_(0) ) )/(-0.693) ]* 1.25\\ln(N)/(N_(0)) = 2.550\\(N)/(N_(0)) = 12.81\\](https://img.qammunity.org/2017/formulas/biology/high-school/myphnj2m3xl3pn9zpodwvwbpbllgnpee9u.png)
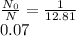
Remaining isotope
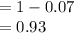
Hence, option D is correct.