Question

Given the equation square root of 2x+1=3 solve for x and identify if it is an extraneous solution

Answer 1

x=4 and I believe it is an extraneous solution, to see if it is extraneous or not

* put x=4 in the original given equation, to see if it satisfies , if not then the solution is not extraneous.

Answer 2

Given the equation:
`√(2x+1) =3` ......[1]

Taking square both sides, we get;

`2x+1 = 3^2`

`2x+1 = 9`

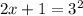
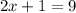
Subtract 1 from both sides we get;

2x = 8

Divide both sides by 2 we get;

x = 4

Extraneous solution states that it is a root of a transformed equation that is not a root of the original equation because it was excluded from the domain of the original equation.

Substitute the value of x in [1] we get;

`√(2(4)+1) =3`

`√(8+1)=3`

`√(9) =3`

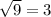
`3 =3` True.

Therefore, the value of x is 4 and it is not an extraneous solution.