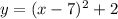Question

The transformations to the parent function of a quadratic equation are given below. Write an equation of the new function in vertex form. Note: ^ denotes "raised to the power of"Transformations: translated 7 units right and 2 units upA y = (x - 7)^2 - 2B y = -(x - 7)^2 + 2C y = (x + 7)^2 + 2D y = (x-7)2 + 2

Answer 1

`y=(x-7)^2+2`

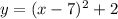
Step-by-step explanation

We want to find the resulting equation when the quadratic parent function is transformed by 7 units right and 2 units up.

The parent function of a quadratic equation is:

`y=x^2`

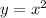
First, let us transform it 7 units right.

This means it is shifting on the x axis. Hence, for different values of x, we have the same values of y.

Transforming the equation, we have:

`y=(x-7)^2`

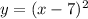
We applied this rule for horizontal translation:

`y=(x-a)^2`

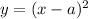
Now, we want to translate it 2 units up.

This means that each x value will now yield a y value that is 2 units greater.

Therefore, we have:

`y=(x-7)^2+2`

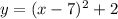
We applied this rule for vertical translation:

`y=x^2+b`

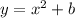
Therefore, the equation is:

`y=(x-7)^2+2`