The solution is the intersection point of the 2 lines.
The given two equations are:
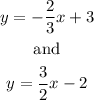
Equating both equations, we can solve for x:

Substituting this value into the second equation, we can find y:
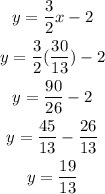
The solution set or intersecting point is:
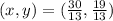
Converting to decimal and rounding we can say:
(x, y) = (2.3, 1.5)