Question

I'm considering the line with the equation y equals x + 9 I need to find a line parallel to it which passes through -2 negative 4 and perpendicular which also passes through negative two negative 4

Answer 1

a) y = x - 2

b) y = -x -6

Step-by-step explanation:
`\begin{gathered} \text{The equation:} \\ \text{y = x + 9} \\ \text{This equation is a staright line and has a slope} \end{gathered}`

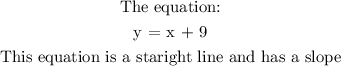
a) parallel line that passes through (-2, -4)

For a line to be parallel to another line, the slope of both lines must be equal

y = x + 9

comparing with equation of line:

y = mx + b

mx = x

m = 1

Slope of first line = 1

slope of the 2nd line = 1

For the 2nd line, it passes through (-2, -4)

x = -2, y = -4

y = mx + b

-4 = 1(-2) + b

-4 = b - 2

-4 + 2 = b - 2 + 2

-2 = b + 0

b = -2

The equation parallel to y = x + 9 becomes:

y = x + (-2)

y = x - 2

b) For a line to be perpendicular to another line, the slope of one line will be thenegative reciprocal of the other line.

slope of 1st line = 1

reciprocal of the line = 1/1 = 1

negative reciprocal = -1

point (-2, -4)

y = mx + b

-4 = -1(-2) + b

-4 = 2 + b

-4 -2 = b

b = -6

The equation perpendicular to the line y = x + 9:

y = -1(x) + (-6)

y = -x -6