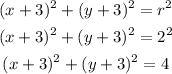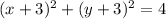
1) Let's start out by locating Center A. A is located at (-3,-3). we can write out the equation of the circle, plugging into that the point (-3, -3)
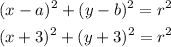
2) Note that we need to find the radius, so let's locate another point in this circle and plug it into the equation above so that we can find the radius. We can locate point (-5,-3) and we can plug into that:
![\begin{gathered} (x+3)^2+(y+3)^2=r^2 \\ (-5+3)^2+(-3+3)^2=r^2 \\ r^2=\mleft(-5+3\mright)^2+\mleft(-3+3\mright)^2 \\ r^2=2^2+0 \\ r^2=4 \\ r=\pm\sqrt[]{4} \\ r=\pm2 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/gzvt4ohvrymi91y82agc.png)
We can discard the negative result, so the answer is:
3)