Question

The area of a rectangular wall in a classroom is 133 ft.². Its length is 2 feet shorter than three times its width. Find the length and width of the wall of the classroom.

Answer 1

`width=7ft;\text{ }length=19ft`

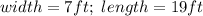
Step-by-step explanation

Let the length of the rectangle be L.

Let the width of the rectangle be W.

The length of the classroom is 2 feet shorter than three times its width. This implies that:

`L=3W-2`

The area of a rectangle is given by:

`A=L*W`

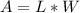
Hence, by substituting the given values into the equation, we have that:

`\begin{gathered} A=(3W-2)*W \\ 133=3W^2-2W \\ \Rightarrow3W^2-2W-133=0 \end{gathered}`

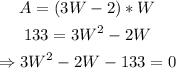
Solve the quadratic equation above by applying the quadratic formula:

`W=(-b\pm√(b^2-4ac))/(2a)`

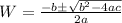
where

a = 3, b = -2, c = -133

Therefore:

`\begin{gathered} W=(-(-2)\pm√((-2)^2-(4*3*-133)))/(2(3)) \\ W=(2\pm√(4+1596))/(6)=(2\pm√(1600))/(6) \\ W=(2\pm40)/(6) \\ W=(2+40)/(6);\text{ }W=(2-40)/(6) \\ W=(42)/(6);\text{ }W=(-38)/(6) \\ W=7;\text{ }W=-(19)/(3) \end{gathered}`

Since the width of a rectangle cannot be negative, the width of the rectangle is:

`W=7\text{ }ft`

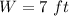
To find the length of the rectangle, substitute the value of W into the equation for L:

`\begin{gathered} L=3(7)-2=21-2 \\ L=19\text{ }ft \end{gathered}`

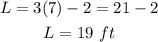
Hence, the width of the rectangle is 7 ft and its length is 19 ft.