Question

One of the factors of the polynomial x3 − 5x2 is x + 3. What is the other factor?

a. x2-8x+24+(72/x+3)
b. x2-8x+24
c. x2-8x+24-(72/x+3)
d. x3-8x2+24x-72

Answer 1

Option C is correct.

Explanation:

Given polynomial : x³ - 5x²

Factors of given polynomial = x + 3

Let, p(x) = x³ - 5x²

To get other factor of p(x), we divide p(x) by given factor.

On division we get,

Quotient = x² - 8x + 24

Remainder = -72

So another factor is
`x^2-8x+24-(72)/(x+3)`

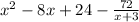
Therefore, Option C is correct.

Answer 2

The answer is letter c. x2-8x+24-[72/(x+3)]. If you do not know how to solve this using the long division method, you can always evaluate the options through the process of elimination first. Since the degree of the other factor is already 1 (x to the power of 1), you know that option d. is not the correct answer because you know that the other factor must be raised to the power of 2. That leaves us with a, b and c. Working backwards and multiplying the given factor (x+3) with the factor in b, gives us x3-5x2+72. So from there, you know that you have to eliminate 72, which can be removed when it is subtracted by itself. Letter c does just that. Try multiplying (x+3) and option c for yourself :).