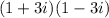Question

BEST ANSWER GETS A METAL!!!!

Which pair of complex numbers has a real-number product?

(1 + 3i)(6i)
(1 + 3i)(2 – 3i)
(1 + 3i)(1 – 3i)
(1 + 3i)(3i)

Answer 1

The answer is (1 + 3i)(1 – 3i).

Answer 2

we will proceed to verify each case to determine the solution

remember that

`i^(2) =-1`

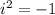
case A)
`(1 + 3i)(6i)`

applying distributive property

`(1 + 3i)(6i)=1*6i+3i*6i \\=6i+18i^(2)\\=6i+18*(-1)\\=6i-18`

`-18+6i` ------> is not a real number

therefore

the case A) is not a real number product

case B)
`(1 + 3i)(2-3i)`

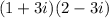
applying distributive property

`(1 + 3i)(2-3i)=1*2+1*(-3i)+3i*(2)+3i*(-3i)\\=2-3i+6i-9i^(2)\\=2+3i-9*(-1) \\=11+3i`

`11+3i` ------> is not a real number

therefore

the case B) is not a real number product

case C)
`(1 + 3i)(1-3i)`

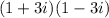
applying difference of square

`(1 + 3i)(1-3i)=(1)^(2)-(3i)^(2)\\=1-9i^(2)\\=1-9*(-1) \\=10`

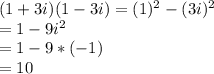
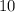`10` ------> is a real number

therefore

the case C) is a real number product

case D)
`(1 + 3i)(3i)`

applying distributive property

`(1 + 3i)(3i)=1*3i+3i*3i \\=3i+9i^(2)\\=3i+9*(-1) \\=3i-9`

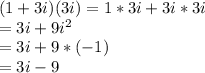
`-9+3i` ------> is not a real number

therefore

the case D) is not a real number product

the answer is

`(1 + 3i)(1-3i)`