1 Answer

Tashima · Answer 1 · 2023-07-17T11:10:50+0000

We know the initial value of the car at time t = 0 and we know that it depreciates 9.7% per year.

If y is the value of the car and t is the time in years, we can write that:

$\begin{gathered} y(t+1)=y(t)-0.097y(t)_{} \\ y(t+1)=(1-0.097)\cdot y(t) \\ y(t+1)=0.903\cdot y(t) \\ (y(t+1))/(y(t))=0.903 \end{gathered}$

This correspond to an exponential decay model, that can be expressed as:

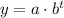
$y=a\cdot b^t$

The parameter a can be calculated as:

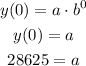
$\begin{gathered} y(0)=a\cdot b^0 \\ y(0)=a \\ 28625=a \end{gathered}$

We can use the previous relationship to find the value of b:

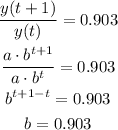
$\begin{gathered} (y(t+1))/(y(t))=0.903 \\ (a\cdot b^(t+1))/(a\cdot b^t)=0.903 \\ b^(t+1-t)=0.903 \\ b=0.903 \end{gathered}$

Then, we can express the value of the car after t years as:

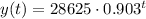
$y(t)=28625\cdot0.903^t$

Then, after t = 10 years, the value is:

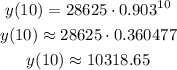
$\begin{gathered} y(10)=28625\cdot0.903^(10) \\ y(10)\approx28625\cdot0.360477 \\ y(10)\approx10318.65 \end{gathered}$

Answer: after 10 years, the value of the car is $10,318.65.

Your comment on this question: