Question

Find the equation of the circle whose center and radius are given.

center ( -2, -5), radius = 1

Answer 1

as i said before put the center instead of (h,k) in the general formula and put r=1
so (x-(-2))^2 + (y-(-5)^2 = 1
(x+2)^2 + (y+5)^2 = 1

Answer 2

Answer: The required equation of the circle is
`x^2+y^2+4x+10y+28=0.`

Step-by-step explanation: We are given to find the equation of the circle with center (-2, -5) and radius of length 1 unit.

We know that

the standard equation of a circle with center (h, k) and radius of length r units is given by

`(x-h)^2+(y-k)^2=r^2~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)`

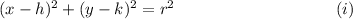
For the given circle, we have

center, (h, k) = (-2, -5) and radius, r = 1 units.

Therefore, from equation (i), we get

`(x-(-2))^2+(y-(-5))^2=1^2\\\\\Rightarrow (x+2)^2+(y+5)^2=1\\\\\Rightarrow x^2+4x+4+y^2+10x+25=1\\\\\Rightarrow x^2+y^2+4x+10y+29-1=0\\\\\Rightarrow x^2+y^2+4x+10y+28=0.`

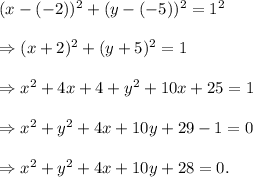
Thus, the required equation of the circle is
`x^2+y^2+4x+10y+28=0.`