Question

There are 29 students in a class . How many ways are there to select 4 students for a group project?

Answer 1

The present case can be determined as follows:

For the first person of the group, there are 29 possible students, for the second person there are 28, 27 for the third, and 26 for the fourth.

It means there would be 29x28x27x26 ways to select students to the group if the order was relevant. But it is not. And it means that, if the group if: Mathew, Arthur, Mary, and Ann, or if it is Ann, Mary Arthit, and Mathew, it doesn't matter at all. The possible way to mix them is 4! = 4x3x2x1

From this, we calculate the number as follows:

`N=(29*28*27*26)/(4*3*2*1)=23,751`

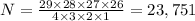
From the solution developed above, we are able to conclude that the solution is 23,751