Question

Identify the vertices, foci and equations for the asymptotes of the hyperbola below. Type coordinates with parentheses and separated by a comma like this (x,y). If a value is a non-integer then type is a decimal rounded to the nearest hundredth. \frac{(y-6)^2}{36}-\frac{(x+1)^2}{16}=1 The center is the point : AnswerThe vertex with a larger y value is the point :AnswerThe vertex with a smaller y value is the point :AnswerThe foci with a larger y value is the point :AnswerThe foci with a smaller y value is the point :AnswerOne of the asymptotes is the equation y=a(x+b)+c Where the value for a is: AnswerWhere the value for b is: AnswerWhere the value for c is: Answer

Answer 1

Given the equation of a hyperbola:

`((y-6)^2)/(36)-((x+1)^2)/(16)=1`

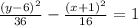
• You need to remember that the equation of a vertical hyperbola has this form:

`((y-k)^2)/(a^2)-((x-h)^2)/(b^2)=1`

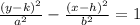
Where the center is:

`(h,k)`

In this case, you can identify that:

`\begin{gathered} h=-1 \\ k=6 \end{gathered}`

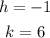
Therefore, its Center is at this point:

`(-1,6)`

• By definition, the Vertices of a vertical hyperbola can be found with:

`(h,k\pm a)`

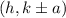
In this case, you know that:

`a=\sqrt[]{36}=\pm6`

Therefore, you can determine that the Vertex with the larger y-value is:

`(-1,6+6)=(-1,12)`

And the Vertex with the smaller y-value is:

`(-1,6-6)=(-1,0)`

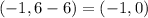
• By definition, the formula for calculating the distance from the Center of a hyperbola to the Foci is:

`c=\sqrt[]{a^2+b^2}`

You already know the value of "a", and you can determine that:

`b=\pm\sqrt[]{16}=\pm4`

Therefore, by substituting values into the formula and evaluating, you get:

`c=\sqrt[]{6^2+4^2}=2\sqrt[]{13}`

By definition, Focis of a vertical hyperbola have this form:

`(h,k\pm c)`

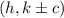
Hence, the Foci with a larger y-value is:

`(-1,6+2\sqrt[]{13})`

And the Foci with the smaller y-value is:

`(-1,6-2\sqrt[]{13})`

• According to the information provided in the exercise, one of the Asymptotes is the equation:

`y=a(x+b)+c`

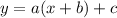
By definition, the equation for the Asymptotes of a vertical hyperbola is:

`y=\pm(a)/(b)(x-h)+k`

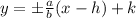
Knowing all the values, you get:

`\begin{gathered} y=\pm(6)/(4)(x+1)+6 \\ \\ y=\pm(3)/(2)(x+1)+6 \end{gathered}`

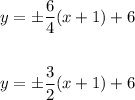
Hence, the answers are:

• Center:

`(-1,6)`

• Vertex with a larger y-value:

`(-1,12)`

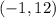
Vertex with a smaller y-value:

`(-1,0)`

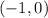
Foci with a larger y-value:

`(-1,6+2\sqrt[]{13})`

Foci with a smaller y-value:

`(-1,6-2\sqrt[]{13})`

Values of "a", "b" and "c" of the equation of one of the asymptotes:

`\begin{gathered} a=(3)/(2) \\ \\ b=1 \\ \\ c=6 \end{gathered}`