Question

Perform the indicated operation and express the result as a simplified complex number. I need help with 35

Answer 1

Given:-

`(3+4i)/(2-i)`

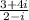
To find:-

The simplified form.

At first we take conjucate and multiply below and above.

The conjucate is,

`2+i`

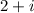
So now we multiply. we get,

`(3+4i)/(2-i)*(2+i)/(2+i)`

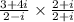
Now we simplify. so we get,

`(3+4i)/(2-i)*(2+i)/(2+i)=(6+3i+8i+4(i)^2)/(2^2-i^2)`

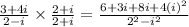
We know the value of,

`i^2=-1`

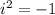
Substituting the value -1. we get,

`\begin{gathered} (6+3i+8i+4(i)^2)/(2^2-i^2)=\frac{6+3i+8i+4(-1)_{}^{}}{2^2-(-1)^{}} \\ \text{ =}(6-4+11i)/(2+1) \\ \text{ =}(2+11i)/(3) \end{gathered}`

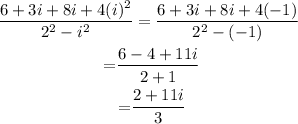
So now we split the term to bring it into the form a+ib. so we get,

`(2+11i)/(3)=(2)/(3)+i(11)/(3)`

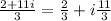
So the required solution is,

`(2)/(3)+i(11)/(3)`