We are asked to find the area of the target outside of the bullseye.
Recall that the area of a circular archery target is given by
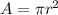
Where r is the radius.
The bullseye has a radius of 3 inches.
The area of the bullseye is given by
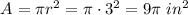
The entire target has a radius of 9 inches.
The area of the entire target is given by
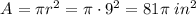
Subtract the area of the bullseye from the entire target area to find the area of the target outside of the bullseye.
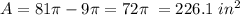
Therefore, the area of the target outside of the bullseye is 226.1 in^2
Option a. is the correct answer.