Question

An unknown number b is 10 more than an unknown number k. The number b is also k less than 8. The equations to find k and b are shown below:

b = k + 10
b = −k + 8

Which is a correct step to find k and b?
Write the points where the graphs of the equations intersect the x-axis. Write the points where the graphs of the equations intersect the y-axis. Add the equations to eliminate k. Multiply the equations to eliminate b.

Answer 1

Add the equations to eliminate k.

Explanation:

When we add the equations we get

`2b =18`

`\boxed{b=9}`

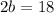
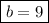
next we put
`b=9` into
`b=k+10` and solve for k:

`9=k+10\\\\\boxed{k=-1}`

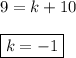
this way we have found k and b; therefore, adding the equations to eliminate k is a correct step for finding k and b.

Let us now look at other choices we were given.

Write the points where the graphs of the equations intersect the x axis.

These points may be the solutions to each equation, but they are not the solutions to the system of these two equations.

Write the points where the graphs of the equations intersect the y axis.

Same thing goes here: these points may be the solutions to each equation, but they are not the solutions to the system of these two equations.

Multiply the equations to eliminate b.

Multiplying the equations doesn't eliminate b, but rather it complicates the matter by producing a quadratic equation—we don't want to go down that road!