Question

How does g(x)=|x-3| -8 compare to its parent function, f(x)=|x|

Answer 1

Given the function g(x) defined as:

`g\mleft(x\mright)=\left|x-3\right|-8`

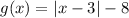
We can go from the parent function f(x) = |x| if we perform the following transformations:

`\begin{gathered} 1.)\text{ }f\mleft(x\mright)\rightarrow f\mleft(x\mright)-8 \\ 2.)\text{ }f\mleft(x\mright)\rightarrow f\mleft(x-3\mright) \end{gathered}`

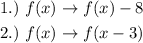
Transformation 1.) is a shift of 8 units down and the transformation 2.) is a shift of 3 units to the right. Then, the graph of g(x) is shifted 8 units down and 3 units right from the graph of f(x).