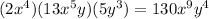Use the laws of exponents and the commutative property of multiplications to rewrite the expression:
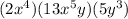
Factor out constants:

Simplify the coefficient of the expression:
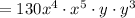
Use the fact that (a^n)(a^m)=a^(n+m) to simplify the powers of the variables x and y:
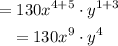
Therefore: