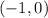Question

First graph and then find the intervals of increasing and decreasing for piecewise function

Answer 1

In order to graph this function, let's identify the type of graph of each part, and then calculate two or more points from each part:

`\begin{gathered} x+2\to\text{ linear} \\ x=-2\colon \\ f(-2)=-2+2=0 \\ x=-3\colon \\ f(-3)=3+2=-1 \\ \\ x^2\to quadratic \\ x=-1\colon \\ f(-1)=(-1)^2=1 \\ x=0\colon \\ f(0)=0^2=0 \\ x=1\colon \\ f(1)=1^2=1 \\ \\ 1\to\text{constant} \\ x=2\colon \\ y=1 \\ x=3\colon \\ y=1 \end{gathered}`

Graphing all these points and the corresponding lines or curves, we have:

Looking at the graph, we can see that the function is increasing for the interval:

`(-\text{inf,}-1)\cup(0,1)`

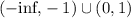
And the function is decreasing for the interval:

`(-1,0)`