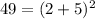Question

What polynomial identity will prove that 49 = (2+5)^2? (A. Difference of Squares; B. Difference of Cubes; C. Sum of Cubes; D. Square of a Binomial)

Answer 1

Hi,

(a+b)² = a²+2ab+b²

For a = 2 and b = 5

(2+5)² = 2²+2(2)(5)+5²
(2+5)² = 4+20+25
(2+5)² = 49

Answer:

D. Square of a Binomial

Answer 2

Option D is correct.

Square of binomials.

Explanation:

Prove that:
`49 = (2+5)^2`

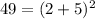
Square of binomials states that the square of a binomial is always a trinomial.

Also, it will be helpful to memorize these patterns for writing squares of binomials as trinomials.

`(a+b)^2 = a^2+2ab+b^2`

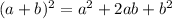
Take RHS

`(2+5)^2`

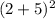
Apply the square of binomial, we have;

`(2+5)^2 = 2^2+2 \cdot 2 \cdot 5 +5^2`

= 4 + 20 + 25 = 24 + 25 = 49 = LHS proved.

Therefore, Square of binomials identity will prove that
`49 = (2+5)^2`