we have
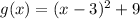
This is the equation of a vertical parabola with vertex at point
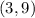
The parabola open upward------> the vertex is a minimum

This is the equation of a vertical parabola with vertex at point
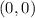
The parabola open upward------> the vertex is a minimum
so
the rule of the translation is

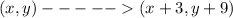
that means
the translation is
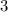 units to the right and
units to the right and
 units up
units up
the graph of the function g(x) is the translated graphic of the function f(x)
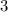 units to the right and
units to the right and
 units up
units up
therefore
the answer is
g(x) is shifted
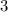 units right and
units right and
 units up
units up