Question

From rest a skier skies decencing on the 30 degree slope .the coefficient of kinectic friction is 0.10

1 ;calculate the:
*acceleration of the skier
*speed of skier after 6.0s

Answer 1

Given:

Slope, θ= 30 degrees

Coefficient of kinetic friction, μ = 0.10

Let's find the following.

(a) Acceleration of the skier.

To find the acceleration of the skier, apply the formula:

`mg\sin \theta-\mu mg\cos \theta=ma_{\text{net}}`

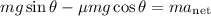
Since we are to find acceleration, rewrite the equation for a:

`a_{\text{net}}=g\sin \theta-\mu g\cos \theta`

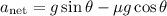
Where:

g is the acceleration due to gravity = 9.8 m/s²

μ = 0.10

θ= 30 degrees

Thus, we have:

`\begin{gathered} a_(net)=9.8\sin 30-0.10\ast9.8\cos 30 \\ \\ a_{\text{net}}=4.9-0.85 \\ \\ a_{\text{net}}=4.05m/s^2 \end{gathered}`

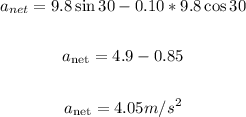
Therefore, the acceleration of the skier is 4.05 m/s².