Answer:
The exponential growth model for the future population is:

The population will be 2266 in 3 years
Step-by-step explanation:
The initial population, P₀ = 1600
The rate, r = 12.3% = 12.3/100
r = 0.123
Time, x = 3 years
The exponential growth model is of the form:
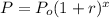
Substitute P₀ = 1600 and r = 0.123 into the equation to get the exponential growth model for the future population
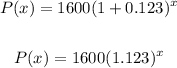
Substitute x = 3 into the model to find the student population in 3 years

The population will be 2266 in 3 years