Question

If EI = 2t + 9 and GI = 9t - 12, find GI in rectangle EFGH.HGIEF

Answer 1

15

Step-by-step explanation:

The Lengths EI and GI are equal; therefore,

`\begin{gathered} EI=GI \\ 2t+9=9t-12 \end{gathered}`

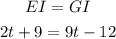
adding 12 to both sides gives

`\begin{gathered} 2t+9+12=9t-12+12 \\ 2t+21=9t \end{gathered}`

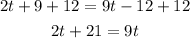
subtracting 2t from both sides gives

`2t+21-2t=9t-2t`
`21=7t`

Finally, dividing both sides by 7 gives

`\boxed{t=3.}`

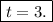
With the value of t in hand, we now find the length GI.

`GI=9t-12`

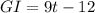
putting in the value of t gives

`GI=9(3)-12`
`GI=27-12`
`\boxed{GI=15}`

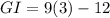
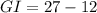
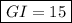
which is our answer!