The Solution:
Given:
A utility pole with a diameter of 18.2 cm and a height of 660 cm. This means that the pole is a cylinder of the form below:
Step 1:
Find the volume of the utility pole.
By formula,

Substituting, we get
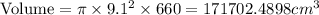
Step 2:
We shall find the mass of the metal used in making the pole.
By formula,
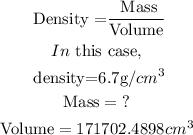
Substituting, we get
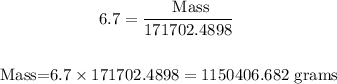
Converting to kilogram, we divide by 1000.
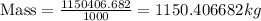
Step 3:
Find the cost.
Given that each kilogram cost $0.87, we have the cost as:
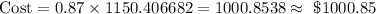
Therefore, the correct answer is $1000.85