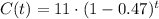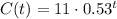Question

Assume an exponential function has a starting value of 11 and a decay rate of ​47%. Write an equation to model the situation.

Answer 1

`C(t)=11\cdot0.53^t`

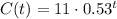
Explanation:

Exponential Decay Function

The exponential function is often used to model natural growing or decaying processes, where the change is proportional to the current quantity.

An exponential decaying function is mathematically written as:

`C(t)=C_o\cdot(1-r)^t`

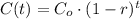
Where:

C(t) is the actual value of the function at time t

Co is the initial value of C at t=0

r is the decaying rate, expressed in decimal

We are given a starting value of Co=11 and a decay rate of r=47%=0.47.

The equation of the model is:

`C(t)=11\cdot(1-0.47)^t`

`C(t)=11\cdot0.53^t`