Question

Which is one of the transformations applied to the graph of f(x) = x2 to produce the graph of p(x) = –50 + 14x – x2?

A.a shift down 1 unit
B.a shift left 7 units
C.a shift right 1 unit
D.a shift up 7 units

Answer 1

Answer: The correct option is (A) a shift down 1 unit.

Step-by-step explanation: The given functions are:

`f(x)=x^2,\\\\p(x)=-50+14x-x^2.`

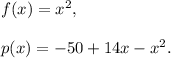
we are given to select the correct option which gives one of the transformations applied to the graph of f(x) to produce the graph of g(x).

We have

`p(x)=-50+14x-x^2\\\\\Rightarrow p(x)=-(x^2-14x+49)-1\\\\\Rightarrow p(x)=-(x-7)^2-1\\\\\Rightarrow p(x)+1=-(x-7)^2.`

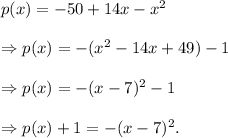
Comparing the equation of p(x) with that of f(x), we conclude that the transformations are

(i) a horizontal shift of 7 units to the right,

(ii) a vertical shift of 1 unit downwards.

So, one of the correct transformations is - a shift down by 1 unit.

The graphs of f(x) and p(x) are attached below.

Thus, (A) is the correct option.