The missing table values include the following;
x 5 -7 -13
y 0 8/3 24
In Mathematics and Geometry, the point-slope form of a straight line can be calculated by using the following mathematical equation (formula):
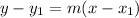
Where:
x and y represent the data points.
m represent the slope.
First of all, we would determine the slope for the line;
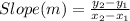
Slope (m) = (8 - 4)/(-1 - 2)
Slope (m) = -4/3
At data point (-1, 8) and a slope of -4/3, a function for this line can be calculated by using the point-slope form as follows:
y - 8 = -4/3(x + 1)
y = -4/3(x) + 20/3
When x is 5, the value of y is given by;
y = -4/3(5) + 20/3
y = -20/3 + 20/3
y = 0
When x is -7, the value of y is given by;
y = -4/3(-7) + 20/3
y = 28/3 + 20/3
y = 8/3
When y is 24, the value of y is given by;
24 = -4/3(x) + 20/3
24 -20/3 = -4/3(x)
52/3 = -4x/3
x = -13