Question

Solve [6x-9]+6<3 how do I solve using interval notation

Answer 1

`\lvert6x-9\rvert+6<3`

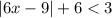
When you have a absolute value in a inequality, to solve:

1. Leave the absolute value in one side of the inequality sing and the other terms in the other side:

-Substract 6 in both sides of the inequatily:

`\begin{gathered} \lvert6x-9\rvert+6-6<3-6 \\ \lvert6x-9\rvert<-3 \end{gathered}`

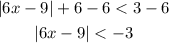
2. As the abosule value is less than a negative number you have no solution for the system, you can see it by following the next steps:

-Write the inequality as two inequalities, one with the sing < and the other with the sing >:

`\begin{gathered} 6x-9<-3 \\ \\ 6x-9>-3 \end{gathered}`

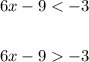
Solve the first inequality:

`\begin{gathered} 6x-9<-3 \\ 6x-9+9<-3+9 \\ 6x<6 \\ (6)/(6)x<(6)/(6) \\ \\ x<1 \end{gathered}`

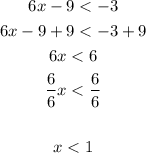
Solve the secodn inequality:

`\begin{gathered} 6x-9>-3 \\ 6x-9+9>-3+9 \\ 6x>6 \\ (6)/(6)x>(6)/(6) \\ \\ x>1 \end{gathered}`

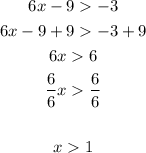
If you combine those solutions you get:

[tex]1As you can see the system has no solution as x cannot be less than 1 and greather than 1 at the same time.

Then, the inequality doesn't have solution