Question

Quadratic formula to find the values of x that are solutions to the equation 2x2 + 3x + 1 = 0

Answer 1

x = -0.5 or -1

Explanation:

Given the quadratic equation;

2x² + 3x + 1 = 0

The standard form of a quadratic equation is ax² + bx + c = 0

Therefore, a = 2, b = 3 and c = 1

Quadratic equation formula is;

`x = \frac {-b \; \pm \sqrt {b^(2) - 4ac}}{2a}`

Substituting into the equation, we have;

`x = \frac {-3 \; \pm \sqrt {3^(2) - 4*2*1}}{2*2}`

`x = \frac {-3 \pm \sqrt {9 - 8}}{4}`

`x = \frac {-3 \pm \sqrt {1}}{4}`

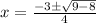
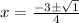
Simplifying further, we have;

`x = \frac {-3 \pm 1}{4}`

`x_(1) = \frac {-3 + 1}{4}`

`x_(1) = \frac {-2}{4}`

`x_(1) = -0.5`

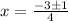
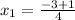
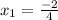
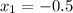
To find the value of x2;

`x_(2) = \frac {-3 - 1}{4} \\x_(2) = \frac {-4}{4} \\x_(2) = -1`

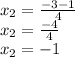
Therefore, the value of x = -0.5 or -1