Question

"find values of a and b that make the statement |a+b|=|a| + |b| false"

Answer 1

Just choose any combination so that a is negative and b is positive, and vice versa. For example, if a = 3 and b = -6, |3-6| ≠ |3| + |-6|.
This is because |-3| = 3, and |3|+|-6| = 3+6 = 9.
3≠9.

Answer 2

a=Any negative number

b=Any positive number

or a=Any positive number and b=Any negative number

Explanation:

We are given that two numbers a and b

We have to find the values of a and b that make the statement
`\mid a+b\mid=\mid a\mid+\mid b\mid` false.

Suppose a=5 and b=-6

`\mid 5-6\mid=\mid 1\mid=1`

`\mid 5\mid +\mid -6\mid=5+6=11`

`\mid 5-6\mid \\eq \mid 5\mid+\mid-6\mid`

`\mid a+b\mid \\eq \mid a\mid +\mid b\mid`

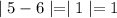
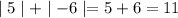
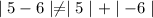
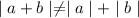
When a be any negative number and b be any positive number or a be any positive number and b be any negative number then it will make given statement false.

Hence, the given statement is false.