Question

A certain vehicle loses 35% of its value each year.18. If the vehicle has an initial value of $25,000, construct a model that represents the value after t years.19. Compute the value of the vehicle at the end of the 3rd year.

Answer 1

`\begin{gathered} A)25000(0.65)^t \\ B)\$6865.63 \end{gathered}`

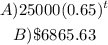
Step-by-step explanation

The general form of an exponential loss is given by:

`A=P(1-r)^t`

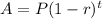
P = initial value of the car.

r = rate of decrease

t = amount of time (in years)

18. Substitute the given values into the equation to find the model that represents the value after t years:

`\begin{gathered} A=25000(1-(35)/(100))^t \\ A=25000(1-0.35)^t \\ A=25000(0.65)^t \end{gathered}`

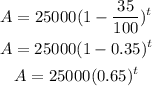
That is the model that represents the value after t years.

19. To find the value of the vehicle at the end of the 3rd year, we have to solve for A when t is 3:

`\begin{gathered} A=25000(0.65)^3 \\ A=\$6865.63 \end{gathered}`

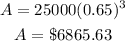
That is the answer.