We have to solve the following
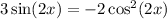
We need to find a trigonometric identity
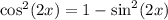
then
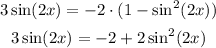
we can rewrite this as
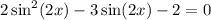
We can make the following substitution
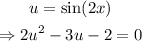
Now, solve with the quadratic formula



So, we get there are two values for u
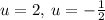
Now, let's substitute back
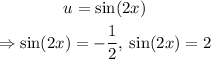
we're almost done!
Now, let's find x for each case
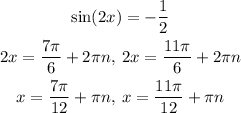
for the other one there is no solution
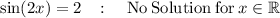
So the answer in degrees from 0° to 360° is:
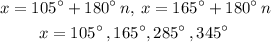
Answer: 105°, 165°, 285° and 345°