Question

A solar sail is a means of spacecraft propulsion using reflected sunlight. The solar intensity is 1380 W/m2. When light is reflected from a reflecting surface it exerts a pressure on the surface of 2*(solar intensity)/(speed of light). If a solar sail of area 43,318.28 m2 and mass 743.39 kg were used to propel a spacecraft, what would its acceleration be in millimeters/s^2? Recall that Pressure=Force/Area.

Answer 1

0.536 mm/s²

Explanation:

Given:

Solar intensity (I) = 1380 W/m²

Area (A) = 43,318.28 m²

Mass (m) = 743.39 kg

Speed of light (v) = 3*10^8 m/s

We can calculate the pressure using the following formula:

`\begin{gathered} P=(2I)/(v) \\ \\ \text{ We replacing} \\ \\ P=(2(1380))/((3\cdot10^8)) \\ \\ P=\:0.0000092\text{ Pa} \end{gathered}`

We can determine the acceleration knowing the following:

`\begin{gathered} P=(F)/(A) \\ \\ F=ma \\ \\ \text{ We replacing:} \\ \\ P=(ma)/(A) \\ \\ a=(P\cdot A)/(m) \\ \\ \text{ We substitute each value to determine the acceleration:} \\ \\ a=(0.0000092\cdot43318.28)/(743.39) \\ \\ a=0.000536\text{ m/s}^2\cdot\frac{1000\text{ mm}}{1\text{ m}} \\ \\ a=0.536\text{ mm/s}^2 \end{gathered}`

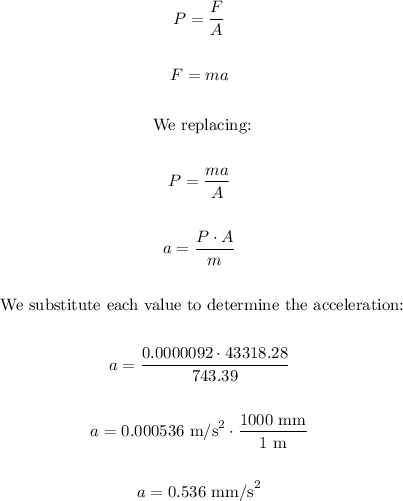
The acceleration is equal to 0.536 mm/s²