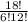Answer:
18564 distinct committee of 12 can be formed
In factorial form:
Explanations:
Note that:

The total number of people, n = 18
The number of people to be selected = 12
The number of distinct committee of 12 people that can be selected from 18 people will be:
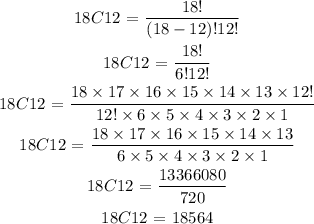
18564 committee of 12 can be formed
This can be written in factorial form as: