Question

Find the equation of the graphUsing y=ax^2+bx+cwrite the equation in vertex form

Answer 1

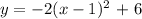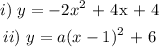
`\begin{gathered} i)\text{ }y=-2x^2\text{ + 4x + 4} \\ ii)\text{ }y=a(x-1)^2\text{ + 6} \end{gathered}`Step-by-step explanation:

i) The equation of parabola is given as:

`y=ax^2\text{ + bx + c}`

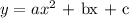
To get the equation of this parabola using the formula above, we will pick any three points on the line. Substitute these points in the formula to get a, b, and c

`\begin{gathered} \text{Using points:} \\ (0,\text{ 4), (1, 6) and (2, 4)} \\ \\ y=ax^2\text{ + bx + c} \\ \text{for (0, 4): x = 0, y = 4} \\ 4=a(0)^2\text{ + }b(0)\text{ + c} \\ 4\text{ = 0 + c} \\ c\text{ = 4} \end{gathered}`
`\begin{gathered} \text{for (1, 6)}\colon\text{ x = 1, y = 6} \\ 6=a(1)^2\text{ + b(1) + c} \\ 6\text{ = a(1) + b + )} \\ 6-4\text{ = a + b} \\ 2\text{ = a + b }\ldots(1) \end{gathered}`
`\begin{gathered} \text{for (}2,\text{ 4)} \\ 4=a(2)^2\text{ + b(2) + c } \\ 4\text{ = 4a + 2b + 4} \\ 4-4\text{ = 4a + 2b} \\ 0\text{ = 4a + 2b }\ldots(2) \end{gathered}`
`\begin{gathered} \text{from equation 1:} \\ a\text{ = 2 - b} \\ substitute\text{ for a in equation (2):} \\ 0\text{ = 4(2-b) + 2b } \\ 0\text{ = 8 - 4b +2b} \\ -8\text{ = -2b} \\ (-8)/(-2)\text{ = }(-2b)/(-2) \\ b\text{ = 4} \end{gathered}`
`\begin{gathered} To\text{ get a, we will substitue for b in equation 1:} \\ 2\text{ = a + b} \\ 2\text{ = a + 4} \\ 2-4\text{ = a} \\ a\text{ = -2} \end{gathered}`

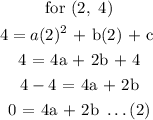
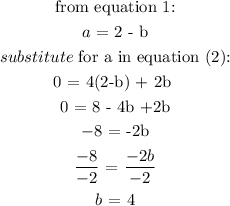
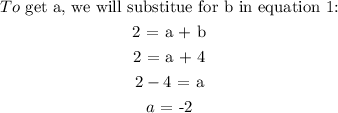
Substittute for a, b and c in the equation we were given

The equation of the graph becomes:

`y=-2x^2\text{ + 4x + 4}`

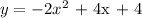
ii) Equation of a parabola in vertex form is given as:

`\begin{gathered} y=a(x-h)^2\text{ + k} \\ \text{where vertex (h, k)} \end{gathered}`

The vertex of the grap is the tip of the parabola. The tip in this case is at x = 1, y = 6

`\begin{gathered} \text{vertex (h, k) = (1, 6)} \\ h=1,k=6 \\ y=a(x-1)^2\text{ + 6} \end{gathered}`

To get a, we will pick a point on the parabola:

`\begin{gathered} u\sin g\text{ y-intercept: vlaue of y when x = 0 } \\ \text{y-intercept: x = 0, y = 4} \\ point\colon\text{ (0, 4)} \\ y=a(x-1)^2\text{ + 6} \\ 4=a(0-1)^2\text{ + 6} \\ 4=a(-1)^2\text{ + 6} \end{gathered}`
`\begin{gathered} 4\text{ = a(1) + 6} \\ 4\text{ = a + 6} \\ 4\text{ - 6 = a} \\ a\text{ = -2} \end{gathered}`

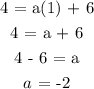
The equation in vertex form becomes:

`y=-2(x-1)^2\text{ + 6}`