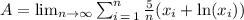Question

Use this definition with right endpoints to find an expression for the area under the graph of f as a limit. Do not evaluate the limit.

Answer 1

To find the expression of the area under the graph f as limit,

Given that,

`f(x)=x+\ln (x),4\leq x\leq9`

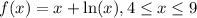
we get,

Consider the small rectangles in the area under the graph f, such that length is f(x) at x point and width is

`\Delta x=(b-a)/(n)`

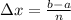
Where a and b are the end points and the n represents the number of partitions.(for n tends to infinity we can get the accurate area under the graph f)

The area of the graph under f is given by,

`\lim _(n\to\infty)\sum ^n_(i\mathop=1)f(x_i)\Delta x`
`=\lim _(n\to\infty)\sum ^n_{i\mathop{=}1}(x_i+\ln (x_i))(9-4)/(n)`
`=\lim _(n\to\infty)\sum ^n_{i\mathop{=}1}(x_i+\ln (x_i))(5)/(n)`
`=\lim _(n\to\infty)\sum ^n_{i\mathop{=}1}(5)/(n)(x_i+\ln (x_i))`

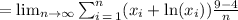
Answer is:

`A=\lim _(n\to\infty)\sum ^n_{i\mathop{=}1}(5)/(n)(x_i+\ln (x_i))`