Question

Graph the line which passes through (6,8) and (9,9). Also state the slope and y- intercept.

Answer 1

First, let's determine the slope and y-intercept of the line to write the equation.

You can calculate the slope of the line using the formula:

`m=(y_2-y_1)/(x_2-x_1)`

Where

(x₁,y₁) are coordinates of one of the points of the line

(x₂,y₂) are the coordinates of the second point

`\begin{gathered} m=(9-8)/(9-6) \\ m=(1)/(3) \end{gathered}`

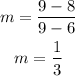
Using the point-slope form you can determine the equation of the line:

Use m=1/3 and (x₁,y₁) as (6,8)

`\begin{gathered} y-y_1=m(x-x_1) \\ y-8=(1)/(3)(x-6) \end{gathered}`

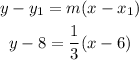
-distribute the multiplication on the parentheses term

`\begin{gathered} y-8=(1)/(3)\cdot x-(1)/(3)\cdot6 \\ y-8=(1)/(3)x-2 \end{gathered}`

-add 8 to both sides of it

`\begin{gathered} y-8+8=(1)/(3)x-2+8 \\ y=(1)/(3)x+6 \end{gathered}`

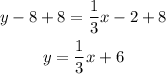
The y-intercept of the line is the constant of the equation b=6

Plot both points and link them to graph the line: