we know that
A rational number is the quotient of two integers with a denominator that is not zero , and can be represented by a repeating or terminating decimal.
Repeating Decimal is a decimal number that has digits that go on forever
Terminating decimal is a decimal number that has digits that do not go on forever.
examples
 (the
(the
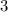 repeats forever)----> Is a Repeating Decimal
repeats forever)----> Is a Repeating Decimal
 (it has two decimal digits)----> Is a Terminating Decimal
(it has two decimal digits)----> Is a Terminating Decimal
therefore
the answer is
A rational number can be written as the ratio of one
 to another and can be represented by a repeating or
to another and can be represented by a repeating or
 decimal
decimal