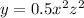Question

Write an equation describing the relationship of the given variables. List variables in alphabetical order. One variable will go within each submission box.

Answer 1

Given that y varies jointly as the square of x and the square of z.

When:

• x = 3

,

• z = 4

,

• y = 72

Let's write an equation describing the relationship of the given varibles.

In this case, we are to make use of the joint variation equation:

y = kx²z²

Where k is the constant of proportionality.

Let's find the value of k.

To solve for k, substitute the given values of the variables into the equation.

We have:

y = kx²z²

72 = 3²×4²×k

72 = 9 × 16 × k

72 = 144k

Divide both sides by 144:

`\begin{gathered} (72)/(144)=(144k)/(144) \\ \\ 0.5=k \\ \\ k=0.5 \end{gathered}`

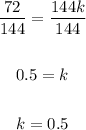
Therefore, the constant of variation, k, is 0.5

The equation describing the relationship will be:

y = 0.5x²z²

ANSWER:

`y=0.5x^2z^2^{}`