ANSWER:
A)
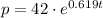
B) week 4
Explanation:
We have that the function has the following exponential form:

A)
We must calculate the value of k, replacing all the corresponding values, like this:
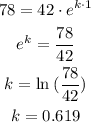
Therefore, the function would be:
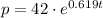
B)
To calculate the time it takes to reach 500, we must plug in the value of p and solve for t, like this:

Which means that the population will reach a population of 500 during week 4