Question

4. The three lines shown in the diagram below intersect at the same point. The measures of some of the angles in degrees are given as 2(-2 + y), 5xº, 36° and 20°. a. Write and solve an equation that can be used to find the value of y. Hint: Use your knowledge of vertical angles.

Answer 1

Part A. Write and solve an equation that can be used to find the value of y.

Given: (Using the principles of vertical angles.)

Solution:

Equation: 2(-2 + y) = 20

`\begin{gathered} 2(-2+y)=20 \\ -4+2y=20 \\ 2y=20+4 \\ 2y=24 \\ (2y)/(2)=(24)/(2) \\ y=12 \end{gathered}`

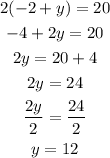
Part B. Write and solve an equation that can be used to find the value of x.

Given: (Using the sum of the angles of a straight line.)

Note: The sum of the angles of a straight line is equal to 180 degrees.

Solution:

Equation: 5x + 36 + 20 = 180

`\begin{gathered} 5x+36+20=180 \\ 5x+56=180 \\ 5x=180-56 \\ 5x=124 \\ (5x)/(5)=(124)/(5) \\ x=24.8 \end{gathered}`

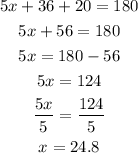
ANSWERS:

Part A: 2(-2 +y) = 20, y = 12

Part B: 5x + 36 + 20 = 180, x = 24.8