Answer:
See explanation.
Step-by-step explanation:
Hello!
In this case, we consider the questions:
a. Ideal gas at:
i. 273.15 K and 22.414 L.
ii. 500 K and 100 cm³.
b. Van der Waals gas at:
i. 273.15 K and 22.414 L.
ii. 500 K and 100 cm³.
Thus, we define the ideal gas equation and the van der Waals one as shown below:
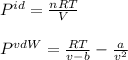
Whereas b and a for hydrogen sulfide are 0.0434 L/mol and 4.484 L²*atm / mol² respectively, therefore, we proceed as follows:
a.
i. 273.15 K and 22.414 L.

ii. 500 K and 100 cm³ (0.1 L).
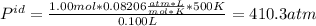
b.
i. 273.15 K and 22.414 L: in this case, v = 22.414 L / 1.00 mol = 22.414 L/mol
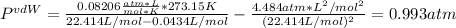
ii. 500 K and 100 cm³: in this case, v = 0.1 L / 1.00 mol = 0.100 L/mol
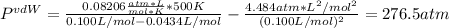
Whereas we can see a significant difference when the gas is at 500 K and occupy a volume of 0.100 L.
Best regards!