The total number of cards in a pack of cards is

The number of face cards in a pack of cards is

The probability of picking one face card is
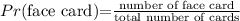

Step 2: We are to pick a second card without replacement
The total number of cards will now be
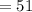
While the number of face cards will now be
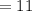
The probability of picking one face card is
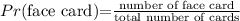
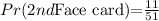
Step 3: We are to calculate the probability of picking the third card without replacement
The probability of picking one face card is
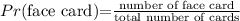
The total number of cards will now be
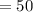
While the number of face cards will now be

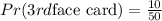
Step 4: We will calculate the probability of picking the fourth face card
The total number of cards will now be
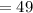
While the number of face cards will now be
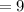
The probability of picking one face card is
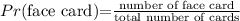
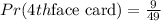
Therefore,
The probability of picking 4 face cards without replacement will be

Hence,
The final answer is= 0.001828