Question

A piano costs $5,000 and loses 20% of its value each year. The table shows the value of the piano after t years.Years Since Purchase (t) Value of Piano (v) 0 $5,000 1 $4,000 2 $3,200 Complete each of the 2 activities for this Task. Activity 1 of 2 Which choice accurately completes the table?A.Years since purchas(t) Value of piano(v) 0. $5,000 1. $4,000 2. $3,200 3. $4,400 4. $5,600B.Years since purchas(t) Value of piano(v) 0. $5,000 1. $4,000 2. $3,200 3. $4,200 4. $5,200C.Years since purchas(t) Value of piano(v) 0. $5,000 1. $4,000 2. $3,200 3. $640 4. $128D.Years since purchas(t) Value of piano(v) 0. $5,000 1. $4,000 2. $3,200 3. $2,560 4. $2,048Activity 2 of 2 What will be the value of the piano after 16 years? Round your answer to the nearest 100. Show your work please!

Answer 1

We know that the piano loses 20% of its value each year, this means $4,000 is 80% of %5,000, $3,200 is 80% of $4,000.

For year 3, we have

`0.80\cdot3200=2560`

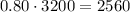
For year 4, we have

`0.80\cdot2560=2048`

Hence, option D is correct.

Let's find the value after 16 years. We know that the ratio of the sequence is 0.80 and the first term is 5,000, let's use the formula for geometric sequences.

`\begin{gathered} a_n=a_1\cdot r^(n-1) \\ a_(16)=5000\cdot(0.80)^(16-1)=5000\cdot(0.80)^(15) \\ a_(16)\approx175.92 \end{gathered}`

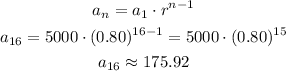
Hence, after 16 years, the value would be $175.92, approximately.