Answer:
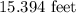
Explanation:
Let
 be the length and width of the base of the prism and,
be the length and width of the base of the prism and,
 be the height of the prism.
be the height of the prism.
Now height of the prism is 2 feet less than the length and width.
So
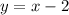
Volume of the the square prism is
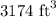
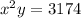
Now we graph both the functions.
The point at which they intersect each other is
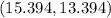
So, the length of the prism is
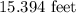 .
.