Answer:
9.48 inches
Step-by-step explanation:
From the diagram given, in order to level the crane, it will have to be raised by x units.
Using trigonometric ratios:
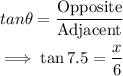
However, since we are required to give our result in inches, convert 6.0 feet to inches.
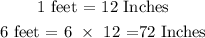
Therefore, we have:
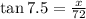
Next, solve for x:
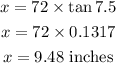
Therefore, the downhill side of the base should be raised by 9.48 inches in order to level the crane.