Answer:
d = 4.59 miles
Explanation:
Distance Between Two Points in the Plane
Given two points A(x,y) and B(w,z), the distance between them is:

The fishing boats leave the marina in perpendicular directions. The first boat travels north at v=12 mph for t=15 minutes. Converting to hours t=15/60=0.25 hours.
The distance covered by this boat is
d1 = 12*0.25 = 4 miles
The boat ends up at the point (0,4).
The second boat travels east at v=9 mph for the same time t=0.25 hours. The distance is:
d2= 9*0.25 = 2.25 miles
This boat ends up at the point (2.25,0)
The distance between them is
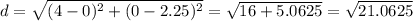
Calculating the square root:
d = 4.59 miles