Question

Let sin(67°) = 0.9205.  Enter the smallest positive value for the angle measure (θ), in degrees, for cos(θ) = 0.9205.

help please

Answer 1

`\cos( \alpha ) = \sin(67) = \cos(90 - 67) = \cos(23)`

Answer 2

θ = 23 degrees

Explanation:

We know that
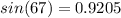`sin(67)=0.9205` (I)

We also know that cos(θ) = 0.9205 (II)

Because the angle 67 degrees is less than 90 degrees we can use the complementary angles equations

The equations are :

cos (θ) = sin ( 90° - θ ) (III)

sin (θ) = cos ( 90° - θ ) (IV)

tg (θ) = cotg ( 90° - θ ) (V)

We can use the equation (III) to find the angle.

If we equalize (I) and (II) :

`0.9205=0.9205` ⇒

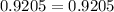cos(θ) = sin(67)

Now, If we use the equation (III) :

cos(θ) = sin(67) = sin ( 90° - θ ) ⇒

67 ° = 90 ° - θ

θ = 23 °

We find that the angle θ is 23 °